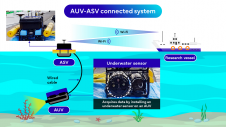
Classroom Exercise

The mathematics a surveyor needs to calculate from measurement-derived quantities such as coordinates, distances, areas and volumes, are taught at length in secondary school, and most people will understand the basics. So surveying can’t be that difficult? Ah, but surveying is not a paper exercise. It is not just a matter of multiplying a given length with a given width to calculate a rectangular-shaped area. Length and width are not givens in the surveying exercise; they have to be measured in a physical environment, either on-site where conditions can be harsh, or in the office using, for example, a Digital Photogrammetric Workstation. A surveyor aims to prevent errors occurring and, once they do occur, to cope with them. And they will occur, not because surveyors are careless, but because measurement errors are an experimental reality.

In his book Principles of Geospatial Surveying (Whittles Publishing CRC Press), A.L. Allan, emeritus reader at University College London, provides a simple but insightful exercise on such errors. Using a 100-mm ruler, each student in the classroom has to draw a straight line 300mm in length. What could go wrong? A lot! The length of lines varies, and this is perceptible by eye-judgement alone. Measuring the lines in centimetres provided the same value each time; measuring in millimetres resulted in varying outcomes. Some rulers gave systematically lower outcomes than others. One student gave the length as 400mm. The exact or ‘true’ value should be 300mm, but only a few students measured this value. This example shows, in a nutshell, all the particularities confronting the surveyor.

A millimetre ruler enables high precision, but at the cost of the introduction of noise; repeated measurements will show slight deviation due to random error. Some rulers systematically provided lower values than others, so measuring equipment needs regular calibration; for example, annually, and this means comparison with a known length which acts as standard. The standard length should have a precision at least ten times better than can be obtained by the device to be calibrated. Finally, one of the students made a blunder. The classroom exercise demonstrates the three types of error that may occur in surveying, namely: random, systematic, and blunders. Nearly every page of Allan’s book is endowed with formulas, most directed towards preventing errors and eliminating them once they occur, as well as quantifying mistakes as a check on the quality of measurements and derived quantities. In other words, a surveyor is no mere wader-wearing chap, but a highly skilled and trained professional.